Maximum Power Transfer Theorem

ByAbragam Siyon Sing Updated on
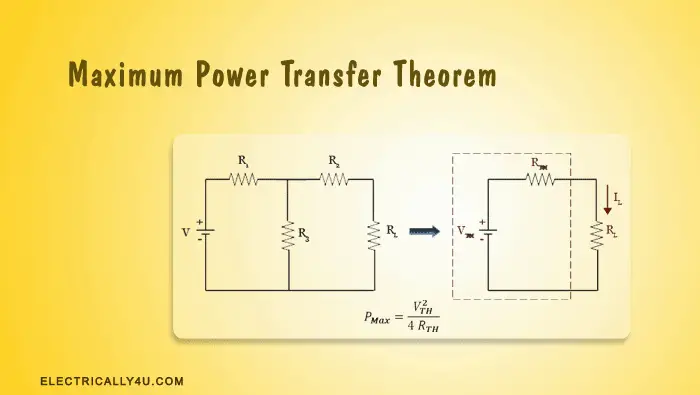
Maximum-power-transfer-theorem

The maximum power transfer theorem is applicable to both AC and DC circuits. In any circuit, the maximum power drawn from the supply depends on the load.

If the load is high, more power is drawn from the supply. Similarly, if the load is less, a small amount of power is drawn from the supply. So, when the maximum power gets transferred to the load? It is clearly explained in this theorem. Let’s learn about this.

The Maximum Power Transfer Theorem states that

“In a linear, bilateral DC network, Maximum Power will be transferred to the load, when the load resistance is equal to the internal resistance of the source.”

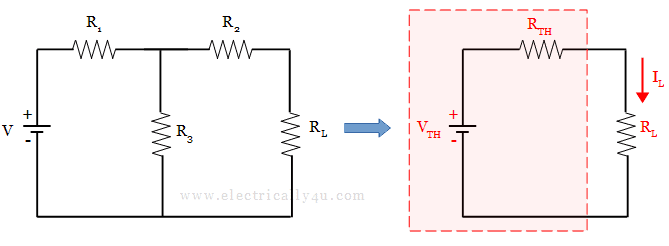
Let us understand the theorem by considering a simple circuit as shown below.

Illustration of Maximum power Transfer Theorem

For the given circuit, apply Thevenin’s theorem to find the equivalent voltage(Thevenin’s voltage) and equivalent resistance(Thevenin’s resistance) of the circuit. Draw the Thevenin’s equivalent circuit as shown.

The power dissipated across the load resistor is given by,

    \[ P_L = {I_L}^2 . R_L ---->(1)\]

The current through the load is given by,

    \[I_L = \frac{V_{TH}}{R_{TH} + R_L } \]

Substitute the above expression in equation (1), we get,

    \[P_L = \left( \frac{V_{TH}}{R_{TH} + R_L } \right)^2 . R_L \]

    \[P_L = {V_{TH}}^2 . \left( \frac{R_L}{(R_{TH} + R_L)^2} \right) ---->(2)\]

Condition for Maximum power transfer

In the above expression, the maximum power delivered depends on the value of load resistance RL. Hence, to obtain the maximum power, the above expression is differentiated with respect to RL and equate it to zero.

    \[\frac{dP_L}{dR_L} = {V_{TH}}^2 .\frac{d}{dR_L}\left[ \frac{R_L}{(R_{TH} + R_L)^2} \right] = 0\]

    \[ => {V_{TH}}^2 . \left[ \frac{(R_{TH} + R_L)^2 * 1 - R_L * 2(R_{TH} + R_L)}{(R_{TH} + R_L)^4} \right] = 0 \]

    \[ {(R_{TH} + R_L)^2 - 2R_L(R_{TH} + R_L)} = 0 \]

    \[ (R_{TH} + R_L)[(R_{TH} + R_L) - 2R_L] = 0 \]

    \[ (R_{TH} + R_L) - 2R_L = 0 \]

    \[ \boxed{ R_{TH} = R_L }\]

The obtained expression is the condition for maximum power transfer. From the expression, we can understand that the maximum power is transferred to the load, when the load resistance is equal to the internal resistance of the circuit.

Substitute RTH = RL in equation (2), we get,

    \[P_{Max} = {V_{TH}}^2 . \left[ \frac{R_{TH}}{(R_{TH} + R_{TH})^2} \right]\]

    \[P_{Max} = {V_{TH}}^2 . \left[ \frac{R_{TH}}{4 {R_{TH}}^2} \right] \]

    \[ P_{Max} = \frac{{V_{TH}}^2 }{4 R_{TH}}\]

Thus the Maximum power transferred to the load is given by,

    \[\boxed{ P_{Max} = \frac{{V_{TH}}^2 }{4 R_{TH}} = \frac{{V_{TH}}^2 }{4 R_L}, Since R_{TH} = R_L }\]

Solved Problem

Find the value of RL at which maximum power is transferred to the load in the following circuit. Also, find the maximum power transferred.

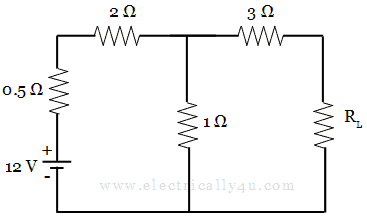
maximum power transfer theorem Problem

We know that the condition for maximum power transfer is, RTH = RL.

Thus to find the value of RL, we need to find the value of Thevenin’s resistance RTH.

To find RTH, follow the steps discussed in Thevenin’s theorem. That is, Short-circuit the voltage source and remove the load resistor. The circuit becomes,

maximum power transfer theorem Problem solution 1

If you observe the circuit, 1 Ω resistor and (2 Ω +0.5 Ω) resistors are in parallel with each other. This parallel combination is in series with 3 Ω resistor. Thus, the equivalent resistance is given by,  

    \[R_{TH} = \frac{1*2.5}{1+2.5} + 3 = 3.714 \Omega \]

    \[R_{TH} = R_L = 3.714 \Omega \]

To find the maximum power that is transferred to the load, we need to find the Thevenin’s voltage. So remove the load resistor in the given circuit and redraw it as below,

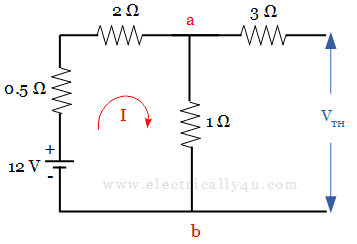
maximum power transfer theorem Problem solution 2

Since the load resistor is removed, the circuit remains open. Since no current flows through the 3 Ω resistor, the Thevenin’s voltage(VTH) is equal to the voltage across a and b(i.e., Vab).

To find the voltage across the ‘a and b’, we need to know the current flowing through it.

So, let us apply Ohm’s Law to the loop,

    \[ I = \frac{V}{R} = \frac{12}{0.5+2+1} = \frac{12}{3.5} = 3.428 A\]

Now, the voltage across 1 Ω resistor(or Thevenin’s voltage VTH) is given by,

    \[ V_{ab} = V_{TH} = I * 1 \Omega = 3.428 * 1 = 3.428 V \]

Therefore, Maximum Power delivered to the load is given by,

    \[P_{Max} = \frac{{V_{TH}}^2 }{4 R_{TH}} = \frac{3.428^2}{4*3.714} = 0.7912 W\]

14 Shares

An Assistant Professor in the Department of Electrical and Electronics Engineering, Certified Energy Manager, Photoshop designer, a blogger and Founder of Electrically4u.

Leave a Reply

Your email address will not be published. Required fields are marked *

This site is protected by reCAPTCHA and the Google Privacy Policy and Terms of Service apply.